Starting from the presumptuous assumption that our interest is primarily focused on particular pieces of music—after all, we never musically listen to anything else—the following statement becomes a useful if controversial characterization: an analytical music theory is a device by which someone communicates his insights about a particular piece of music. We can then expect an orgy of creation of theories, since it is unreasonable to expect that a theory carefully tailored for one piece would fit many others equally well, without being Procrustean. Granted, certain categories of pieces have arisen, like the "tonal" or the "twelve-tone serial," such that for each category a single theory may serve to express useful kinds of things—that is, certain useful relations—which, therefore, the pieces within that category "have in common." But to describe adequately the relations that constitute, for example, my hearing of Brahms' Opus 116 number 6, it is necessary to employ a theory which differs significantly from the tonal theory of (for example) Heinrich Schenker, while retaining much in common with that more generally and less specifically tailored theory.

Our intent is not to decry such generally tailored theories, which at the very least may provide initial approaches to a particular piece, but to explore the means by which we can express precisely what these and other created theories are.

In order to communicate, a music theory must be intelligible. Fortunately, there is a calculus of intelligible declarative statements, called formal logic. Formalization of a theory within this predicate calculus can expose weaknesses in the means of expression. It does not provide any judgments about that which is expressed. However, a clarification in means of expression may strip the Emperor's new clothes from an always unsatisfactory but previously obfuscated concept.

In making theories, to form a concept is usually to form a definition. A flawed definition is a flawed concept. The music-theoretical literature is a porridge of definitions fallen prey to various dangers, and worse, of "definitions" so-called which are utterances of every possible sort except the sort of definition. If only authors who cannot define would confine themselves to the "you know what I mean" mode of discourse, all would be well. But a statement falsely purporting to define, if taken seriously, utterly destroys the fabric of its context.

For some of the dangers of definitions and for rules for constructing definitions, I refer you to Benson Mates' Elementary Logic, pages 197-203, or to any good textbook on mathematical logic or axiomatic set theory (see Bibliography). Very briefly, a definition must be both eliminable and non-creative. It is "eliminable" if the defined expression may be replaced by the defining expression in all contexts without change of truth value. It is "non-creative," in this technical sense, if it generates no new theorems in which the defined expression does not occur. For example, a certain definition of "interval-class" is "creative" because from it can be deduced theorems such as 3 = 5 (mod 12); this definition is inconsistent and therefore "creative." Basically, the defined expression must be nothing more than an abbreviation for the defining expression. The act of defining is certainly "creative" in the colloquial sense, singling out or constructing one out of an infinity of possible expressions and abbreviating it for convenient use toward some purpose.

Formalization is attractive for aesthetic considerations as well as utilitarian ones. Any music theory—any theory of any sort, in fact—can be constructed as an extension of an axiomatic set theory. An axiomatic set theory is itself merely a first-order predicate calculus with the addition of a primitive two-place predicate, which is read "x is an element of y" under standard interpretation, and a minimum of three axioms (four, if you need infinity). To such a set theory, add a few musical primitive predicates and axioms, and you have the foundation of whatever music theory you may want. Can anyone resist admiring the beautiful economy and scope of such a formulation?

All the definitions that follow are constructed or constructible within a formal theory that is an axiomatic set theory with the addition of two primitive predicates, which are read "x is a pitch" and "x is a time" under standard interpretation. For all practical purposes, the set theory assumed here is a medium strong Zermelo-Fraenkel set theory. A stronger set theory, whose axioms include not only the axiom of extensionality and the axiom schema of separation, but also the axiom of infinity, the axiom schema of replacement, the axiom of choice, and the axiom of foundation—such a theory is a foundation for almost all of mathematics, and certainly for all the number theory and other miscellaneous mathematics found convenient in music theory. But music theory might well get away with only a mathematics of (perhaps very large) finite numbers; and the axiom of choice and others are unnecessary without the axiom of infinity—a tempting simplification.¹

Among the less interesting consequences of axiomatic set theory is the Boolean algebra of set union and intersection, which is sometimes referred to as "set theory" in the music-theoretical literature. For those of you who are interested in pursuing axiomatic set theory, I recommend a book called Foundations of Set Theory, by Fraenkel, Bar-Hillel, and Levy. The production of various theorems of axiomatic set theory and mathematics is hereby assumed.

We will also assume the development of a fundamental part of the formal music theory that is an extension of axiomatic set theory. Such a development defines and enumerates twelve equal tempered adjacent ascending pitches per octave, that is, interprets the relation of "x is a pitch one semitone higher than y" as modelled by a particular relation of "c is an integer immediately succeeding b."² This can be a very problematic (and interesting) development, considering the non-transitive nature of a perceptual relation such as Boretz's "pitch matching" (in Benjamin Boretz's Meta-Variations), and also considering whether a desirable ontological parsimony would allow a domain of distinguishable pitches that is, in order of preference, either finite, denumerable, or a continuum. But, "you know what I mean," or you can read and ponder Boretz, or indeed I could present an alternative foundational theory to Boretz's in a separate paper. For the time being, merely consider the 88 keys of the piano, numbered upwards, and let them serve (as a segment of an enumeration) to enumerate the pitches they represent and any lower or higher pitches. We assume also a similar development (even more controversial) of a fundamental theory of perceived times-within-a-piece.

In a previous paper³ I have presented a tentative system of about fifty successively dependent fully formalized definitions and definitional schemata for predicates and operations that are basic to most theories of music using equal temperament, starting with a definition of "the ordered pitch interval between x and y" and ending past such beauties (or beauts) as number 35 on that list, a definitional schema for "ordered/unordered pitch/pitch-class interval line equivalence."⁴ But this present paper angles toward a different, tonal-theoretical illustration of the methodology that is its message. A recapitulation of the previous system would take us off the track.

I will instead casually observe that the terms defined in this previous, more basic system were either operations or predicates; that every n-place operation can be (and often was) redefined as a (n + 1)-place predicate; that the words "predicate" and "relation" are interchangeable here; that the expression defined and the expression defining it are always connected with an "if and only if" biconditional; that all free variables must be universally quantified over the entire definition; and that equivalence classes of many different sorts seemed to be very useful things to define. Moreover, there are many traps for the unwary, or even the wary, who attempts to formalize even the most familiar of concepts (such as that of pitch-class interval). I discovered that many old friends had been clothed in Imperial garments, and were in desperate need of physical therapy.

In place of that system of fully formalized definitions, I offer here a system of definitions expressed in English in such a way that their formalization should pose no problems in principle; after all, this latter is as formal a mode of discourse as most of us ever find opportunity to use. While the first, formalized, unpresented system dealt mainly with more basic concepts and with concepts pertinent to non-tonal music, this second, less formally presented system is a rudimentary but complete theory for tonal music. Its virtues are that it is rudimentary and therefore extendible into particular more detailed theories tailored for specific pieces, and that even its rudiments are easily modifiable, with specific effects. Moreover, it is a theory of both pitch and rhythm. "Level analysis" is defined in this theory, as a predicate, and examples of level-analyses satisfying this definition will be given. Every level in such an analysis is expressible in unmodified musical notation, and thus may be performed—on a piano, for example. This kind of direct connection between the analytical and the audible is invaluable.

This theory is, again, rudimentary, and is by no means my candidate for a really satisfactory tonal theory. But, being an oversimplification, it can quickly be taught in all its rigor to lower-division undergraduate music students; being rigorous, it provides them with a discipline which enables them later to grasp more sophisticated and/or obscure and ambiguous Schenkerist theories with (in my experience) very little effort and confusion. Such theories include, besides Schenker's, Peter Westergaard's, Arthur Komar's, and Maury Yeston's, Narmour's recent constructive polemic, and Lerdahl and Jackendoff's article, to mention only a few in this burgeoning field (see Bibliography).

There does exist an evolving enriched version of the theory presented here, a version that attempts to express also subtler intuitions, especially in regard to the integration of the degree of backgroundness of pitch/timepoints with the music's perceived development to an arrival, in a sense of "arrival" that counterpoints metrical and non-metrical structures. Also of great interest to me is a radically different theory—not an extension of this paper's theory—which retains a sense of syntactical and structural hierarchy while focusing almost exclusively on the (Schenkerian far foreground) motivs. Medieval music has been particularly influential in the development of this theory.

After this reminder of the desirability of an attitude of pluralism toward musical theories, let us return from the voyeuristic contemplation of the larger orgy to the closer pursuit of the particular nymph at hand.

The definitions that constitute the theory will be given in a natural order; earlier definitions are used in later definitions. For each numbered definition, there may be several alternatives labelled with letters, such as VA, VB, and VC. In fact, this is rather a Chinese menu of a theory. Various different theories, of which this total theory is an extension, may be constructed by choosing one or more lettered alternatives from each numbered definition.

We begin with two very basic definitions, of "note" and "rest," which would seem quite guileless. However, I experimented with not defining "rest," assuming that rests could be accounted for as the mere absence of notes. This can be done, but only at the price of some less straightforward definition of "arpeggiation" later.

I	x is a note	IFF x = < z, < T1, T2 >> for some value of z, T1, T2.
II	x is a rest	IFF x = < s, < T1, T2 >> for some value of T1 and T2 (s is a constant).

Under standard interpretation, "z" in the definition of "is a note" takes values that are pitches, "T1" values that are times of initiation and "T2" values that are times of termination, or "release times," with time zero being the beginning of the piece under consideration in an arbitrary or imaginary performance. A "rest" is defined as a note whose pitch is the constant "s" (for silence).

III

x and y are

time-adjacent IFF x and y are notes or rests and T2 of x equals T1 of y or T2 of y equals T1 of x. (One note begins where the other leaves off.)

Definitions IVA and IVB are crude and simple, but effective, particularly when presented to an audience (such as lower-division undergraduates) some of whom might fear and therefore resent the sophistication of a more refined version of these definitions.

IVA	x and y are	pitch-adjacent IFF x and y are notes whose pitches are a minor, major, or augmented second apart.
IVB	x and y are	circle of fifths pitch-adjacent IFF x and y are notes whose pitches are a perfect fourth or fifth apart.

Both of these—IVA and IVB—may be seen to be special cases of a much more powerful definition such as IVC. The idea of so using adjacency within an ordered "syntactic" collection, C, is very reminiscent of Benjamin Boretz's Meta-Variations.

IVC

x and y are

pitch-adjacent with respect to C IFF C is a cyclic ordering of pitch-classes and x and y are notes whose pitches are less than an octave apart and belong to pitch-classes that are adjacent in C.

Definition IVC is really a "definitional schema." The individual definitions IVD through IVH result from substituting particular values for the variable "c" in definition IVC, values that are "syntactical" collections useful for mainstream tonal music. Definition IVC is nicely flexible: for Debussy, the variable "c" may usefully become the whole-tone scale and the (0-4-8) trichord; for Scriabin, the octatonic scale and the (0-3-6-9) tetrachord, etc.

IVD	x and y are	chromatically adjacent IFF x and y are pitch-adjacent with respect to the chromatic scale.
IVE	x and y are	diatonically adjacent IFF x and y are pitch-adjacent with respect to a major scale.
IVF	x and y are	extended diatonically adjacent IFF x and y are pitch-adjacent with respect to a major or harmonic minor or melodic minor scale.
IVG	x and y are	circle of fifths adjacent IFF x and y are pitch-adjacent with respect to the circle of fifths.
IVH	x and y are	triad-adjacent IFF x and y are pitch-adjacent with respect to any (cyclic) ordering of a major or minor pitch-class triad.

Now the sense can be seen in which IVA and IVB are crudely expressed special cases of IVC: x and y are pitch adjacent (definition IVA) if they are chromatically adjacent (definition IVD) or extended diatonically adjacent (definition IVF); and x and y are circle of fifths pitch adjacent (definition IVB) if and only if they are circle of fifths adjacent (definition IVG).

In definition IVC, the words "are less than an octave apart and" may be deleted to leave a definition that would allow, in my opinion, a much too promiscuous use of "octave transfer" type operations, but then, in my present opinion, the fewer of these kinds of operations the better. If one must allow octave transfer, and it seems that one must, it is better to introduce it later, separately, for use in highly circumscribed conditions only.

Terms that are used in IV that have not been defined are legion: "a minor/major/augmented second apart," "a perfect fourth/fifth apart," "an octave apart"; "scale," "the chromatic scale," "a major scale " "an harmonic minor scale," "a melodic minor scale," "the circle of fifths," "a major triad," and "a minor triad." That's a lot of terms. All of these must be defined before any expressions in which they appear will make sense. "Pitch-class" was defined in the previous system of definitions not given here, and "cyclic ordering" and "adjacent in a cyclic ordering" are defined in set theory. By implication from IVC, "the chromatic scale," "a major scale," "an harmonic minor scale," "a melodic minor scale," and "the circle of fifths" are each a "cyclic ordering of pitch-classes" of some sort. Exactly what sorts of cyclic orderings they are, may be defined using my (unpresented here) definitions (or anyone's definitions, actually) of "pitch-class," "pitch-class ordered interval," etc. Major and minor triads were defined under other names in that unpresented system.

Finally we come to a definition of neighbor-note, for which the previous definitions of time adjacency and pitch adjacency are ancillary. Three alternative definitions are given. Definition VA incorporates the crude but superficially easy definition of pitch adjacency, IVA. Definition VB incorporates IVB or its equivalent IVG; and VC incorporates the much more powerful and sophisticated definition, IVC, of pitch adjacency with respect to a reference collection, C.

VA	x and y are	neighbors IFF x and y are time-adjacent and pitch-adjacent (IVA).
VB	x and y are	N* neighbors IFF x and y are time-adjacent and circle of fifths pitch-adjacent (IVB) or circle of fifths adjacent (IVG).
VC	x and y are	neighbors with respect to C IFF x and y are time-adjacent and pitch-adjacent with respect to C (IVC).

In a surprisingly short time we are ready, now, to make formalizable definitions of such high-falutin' and often fatally ambiguous concepts as prolongation, background, level-analysis, and level. These will not be identical to the orthodox Schenkerian concepts (insofar as those can be surely defined) but do correlate closely with them in many ways.

Definitions VIA, VIB, and VIC, differing only in their first clause, are alternative definitions of neighbor-note prolongation incorporating, respectively, the above definitions of neighbor-note, VA, VB, and VC. Definition VI reads:

VIA	x and y	N-prolong z IFF x and y are neighbors and z is a note whose pitch equals the pitch of x or of y and whose initiation (value of T1) is the earliest initiation of x or of y and whose release (value of T2) is the latest release of x or of y.
VIB	x and y	N*-prolong z IFF x and y are N* neighbors and z is a note whose pitch equals the pitch of x or of y and whose initiation (value of T1) is the earliest initiation of x or of y and whose release (value of T2) is the latest release of x or of y.
VIC	x and y	NC-prolong z IFF x and y are neighbors with respect to C and z is a note whose pitch equals the pitch of x or of y and whose initiation (value of T1) is the earliest initiation of x or of y and whose release (value of T2) is the latest release of x or of y.

Examples 1 and 2 show some notes x, y, and z that fulfill definition VIA. In each case, z must occupy the combined timespans of x and y; that is, the T1 of z is the T1 of x and the T2 of z is the T2 of y in these examples.

 

Ex. 1

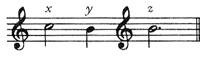
Ex. 2

 

The next definition, VII, is only one of many possible definitions of prolongation by arpeggiation.

VII

A

arp-prolongs B IFF A is a set of notes or rests and B is a set of notes and a pitch is in A IFF it is in B, and all initiations (T1) in B are equal to each other and equal to the earliest initiation in A, and all releases (T2) in B are equal to each other and equal to the latest release in A.

Example 3 shows one pair of sets, such that the set labelled "A" arp-prolongs the set labelled "B," according to definition VII. Again, T1 of B equals T1 of the first sixteenth-rest of A, and T2 of B equals T2 of the last sixteenth-rest of A.

 

Ex. 3a

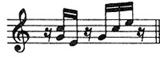
Ex. 3b

 

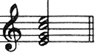
All useful definitions of arpeggiation that I've been able to think up share the characteristic of providing a much less determinate relation among their terms than does a definition of neighbor-note prolongation. If x and y N-prolong z, as defined in VIA, then for any given values of x and z, y is either uniquely determined (if the pitch of x is not equal to the pitch of z) or is determined to within one of two notes of identical duration and of pitches adjacent on either side of the pitch of z. Also, for any given values of x and y, z is determined similarly to within one of two notes. For this particular definition of arpeggiation (VII), if A arp-prolongs B, then a particular value of A uniquely determines B; but, for any given value of B, there is an arbitrarily large number of values for A—not to say infinite. In other useful definitions of arpeggiation, a particular value of A does not even uniquely determine B.

Definition VIII defines, in effect, what it is to be adjacent levels, although "level" as such is not defined until definition X.

VIII

A

is a next-background to B IFF A and B are distinct sets and for at least one set A1 and at least one set B1, A1 partitions A and B1 partitions B and there is at least one one-to-one correspondence, X, from A1 to B1, such that for every member of X, < a,b >, b = a or b NC-prolongs a or b arp-prolongs a.

Since this has proven to be a slippery definition, its formalization is included.⁵

 

Ex. 4a

Ex. 4b

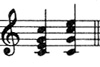
Ex. 4c

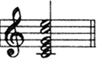
Ex. 5a

Ex. 5b

Ex. 5c

 

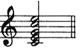
Example 4b is a "next-background" (as defined in VIII) to Example 4a, and 4c is a next-background to 4b. Examples 5a, 5b, and 5c are identical to 4a, 4b, and 4c, with the addition of some diacritical marks. These marks are totally redundant—they provide no information not provided by the set of levels viewed in the light of the definitions. The diacritical marks serve to reinforce the perception of the relations displayed by example 4 and the theory, and thus may be at least pedagogically useful.

The definitions of "level-analysis" (definition IX) and of "level" (definition X) follow relatively easily from the above.

IX	A	is a level-analysis of B IFF B is a set of notes or rests and A is a set of sets of notes or rests and B is an element of A and every member of A except B is a next background to exactly one member of A.
X	A	is a level IFF for some value of X and Y, A is an element of X and X is a level-analysis of Y.
	(A	is a level if A is a member of some level-analysis.)

In fact, Examples 4a, 4b, and 4c together constitute one possible level-analysis of Example 4a according to definition IX. Example 6a equals Example 4a, but Examples 6a through 6f constitute a quite different level-analysis of it than Example 4, although still fulfilling definition IX.

 

Ex. 6a

Ex. 6b

Ex. 6c

Ex. 6d

Ex. 6e

Ex. 6f

 

Before illustrating this theory further with particular level-analyses (which fulfill definition IX), I'd like to point out yet another crucial difference between the two operations of the system. (They are actually defined as predicates, not as operations, but remember that any n-place predicate may be redefined trivially as an (n 1)-place operation.) Moving toward the foreground, both neighbor note and arpeggiation produce new timepoints. But only neighbor note produces new pitches, and those one at a time. Moving toward the background, neither operation produces new pitches or new timepoints. This means that any pitch or timepoint generated at some level will be present in all more foreground levels. In fact, one can quantify the "degree of backgroundness" of a pitch or a timepoint in a particular level-analysis by counting the levels in which the pitch or time is present. "Degree of backgroundness" thus might be used in building a more sophisticated theory, that includes a theory of meter.

A simple but flexible little theory is achieved by limiting NC-prolongation (in VIII) to N-prolongation (VIA, VA, IVA) and N*-prolongation (VIB, VB, IVB), where N*-prolongation is further restricted in application to the lowest pitches in some context; that is, circle-of-fifths neighbor-prolongation is restricted to the bass. The attached level-analysis of measures 1-4 of Mozart's Sonata K. 331 satisfies definition IX under the above restrictions, where the "A" of definition IX is the set of levels shown and the "B" is the most foreground level, here equal to the score. Notes and rests are given in normal musical notation.

 

Ex. 7: Mozart, K. 331, measures 1-4 (1-8).

 

This is only one of very many analyses of this Mozart that satisfy definition IX, as should be apparent from a comparison of Examples 4 and 6. This is not the place in which to discuss the intuitions by which this particular analysis was arrived at, or the ways in which analytical decisions in this theory closely reflect decisions in performance and in listening. I do enjoy hearing the piece through this analysis, but would be even happier with a plurality of analyses, and am always open to persuasion.⁶

Secondly, this analysis not only satisfies the generally tailored definition IX, but also a much more specific definition produced by adding clauses to definition IX; we shall discuss a few such clauses later. For the present, I will briefly discuss some of the rhythmic-motivic inter-level structures that contribute coherence to this analysis; similar pitch-motivic structures should be relatively obvious. The principles of these particular kinds of pitch and rhythmic inter-level structures might eventually be added to definition IX as part of the theory for and of this piece.

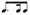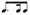In level 3, measure 4, a rotation of the rhythm of level 1 appears in the upper voices, and in the bass here appears a rotation of the rhythm of levels 1 and 2. In level 4, measures 3-4, the rhythm of levels 1 and 3 reappears; level 5 will partition this rhythm, in the bass, into two halves, 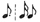, as level 2 partitioned the 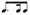of level 1. The 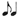, which appeared in the bass in level 3, measure 4, also appears, rotated again, in the non-bass voices in level 5, measures 3-4. Finally, the 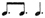rhythm predominant in level 2 reappears at level 7, as measures 1-2, 3-4, and 5-8, linking the far foreground with the far background. Also in level 7, the in measure 4—the leading tone of the dividing dominant of measures 1-8—cannot, in this theory, prolong the tonic of measures 1-3, letting us know that the piece is unfinished without measures 5-8.

Returning to definition IX for a moment, here are four clauses that can be added to definition IX to produce theories that are more specific, although still rather general:

1.	. . . and	the most background level contains only one T1 and one T2
2.	. . . and	for any timespan within some level that contains only pitches that belong to a certain diatonic collection, some more background level must contain only pitches in the tonic triad of the diatonic collection within that timespan
3.	. . . and	the most background level of A must contain no pitches other than those of a (root position) major or minor triad
4.	. . . and	some level of A contains only the triads I-V-I in that order, embedding a Schenkerian Ursatz.

If all four clauses were added to definition IX, the analysis of measures 1-8 of the Mozart would still satisfy the definition. Various terms, such as "Schenkerian Ursatz" and "most background," would have to be defined in this system in order for these clauses to make sense. Clause number 2 implies clause number 3. More clauses can and should be added for specific effect. Operations such as "octave transfer," "passing note," and "upbeat prolongation" may also be defined and added to the theory.

This paper has been rather gung-ho on definitions, but there are other ways to formalize a music theory. In fact, following Michael Kassler—at a distance—I have in the past tended to express my music theories as independent axiomatic or deductive systems, in which relations like neighbor-note prolongation become transformation rules and things like Schenkerian Ursätze become axioms; each level of any analysis becomes a theorem of such a theory. Of course, transformation rules, axioms, etc., must still be defined, but outside the theory rather than within it.

It's fun to create theories that can express precisely how a piece is heard. The foregoing has been intended to indicate at least one way in which this theorist is learning to rend his garments of dull ambiguity.

 

BIBLIOGRAPHY

Bernays, Paul. Axiomatic Set Theory. Amsterdam: North-Holland, 1968.

Boretz, Benjamin. Meta-Variations: Studies in the Foundations of Musical Thought. Diss. Princeton 1970. Published serially in Perspectives of New Music: Fall 1969, pp. 1-75; Spring 1970, pp. 49-112; Fall 1970, pp. 23-42; Spring/Fall 1971, pp. 232-270; Fall 1972, pp. 146-223; Spring 1973, pp. 156-203.

Fraenkel, Abraham, Yehoshua Bar-Hillel, and Azriel Levy. Foundations of Set Theory. Amsterdam: North-Holland, 1973.

Kassler, Michael. A Trinity of Essays. Diss. Princeton 1968.

Komar, Arthur. Theory of Suspensions. Princeton: Princeton Univ. Press, 1971.

Lerdahl, Fred, and Ray Jackendoff. "Toward a Formal Theory of Tonal Music." Journal of Music Theory, 21.1 (1977), pp. 111-171.

Mates, Benson. Elementary Logic. 2nd ed. New York: Oxford Univ. Press, 1972.

Narmour, Eugene. Beyond Schenkerism. Chicago: Univ. of Chicago Press, 1977.

Westergaard, Peter. An Introduction to Tonal Theory. New York: W.W. Norton, 1975.

Yeston, Maury. The Stratification of Musical Rhythm. New Haven: Yale Univ. Press, 1976.

---

¹The three axioms sufficient for set theory are 1) extensionality 2) aussonderungsaxiom 3).

See Foundations of Set Theory by Fraenkel, Bar-Hillel, and Levy, pp. 44-45, and its references. A few more axioms make a handier set theory but are eliminable. The axiom of infinity and the axiom of choice, on the other hand, each extend the set theory, as do certain other possible axioms.

²See Meta-Variations by Benjamin Boretz. In this paper "x is a pitch" corresponds to Boretz's defined predicate "x is a pitch-function."

³"Away From Some Non-Definitions: Logic, Set Theory, Music Theory: I." Presented at a University of Washington Music Colloquium, April 15, 1977. Despite the title, this paper was different in substance from any version of the present essay.

⁴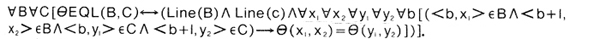
Greek letters are meta-linguistic place-holders with restricted ranges within the set of previously defined terms, making this a definitional schema. All predicates and operations in defining expressions were previously defined.

⁵Formalized, definition VIII reads: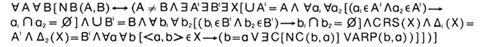

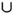"B¹" designates the union of all elements of B¹.
"Crs(X)", or, "X is a one-to-one correspondence", is defined: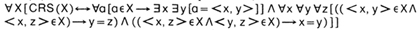

The above and other ancillary definitions (such as 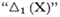) are adapted from Bernays, Axiomatic Set Theory, especially pp. 60-61; see 3.11, 3.12, 3.13, 3.14, and 4.3.

⁶I am indebted in this footnote to Mr. Haflich (of Yale) who (during public discussion of this paper as read to the Second National Conference on Music Theory at Evanston, Illinois, Nov. 18, 1977) characterized this analysis as "wrong." By me, an analysis that violates the syntax of its theory is "wrong" (assuming that the theory under which the analysis operates is well-defined enough to admit of violation). I believe this analysis does not violate the syntax of the stated theory, and is therefore, by my lights, "right." However, a "correct" analysis may still be ugly, quirky, or otherwise repugnant, under my theory, since the theory does not attempt to incorporate explicitly criteria that would make all "ugly" analyses non-syntactical. I am aware that this analysis may well seem at least quirky, even ugly, and could conceivably be myself convinced of its ugliness. During further private discussion with Mr. Haflich, there arose before me the notion that a theory should be so constructed that any "correct" analysis in that theory will be a pretty analysis, maybe even the one unique most beautiful analysis. In this case, all other theories would produce uglier analyses; but The Theory would produce a unique, correct, and absolutely beautiful analysis for each piece. Run the other way, generatively, this Theory would produce only music of unsurpassable beauty; perfect music. My mundane theory could generate some terrifically ugly music, just as it admits of correct but ugly analyses. All music theories I know of also can generate ugly (unwanted) music and admit syntactically correct but ugly (unwanted) analyses. For the time being, I am content to try to construct theories that are capable of expressing (or generating) beauty in music. To attempt to bias theories toward the expression/generation of beautiful (wanted) kinds of structures is a constructive and ongoing strategy. But to attempt to make a theory generate all and only beautiful music, a theory which would be capable of delivering (syntactically) only the one most beautiful analysis, seems to me futile. One man's meat is another man's poi.