In presenting to students the materials of his discipline, the musicologist normally has recourse to words.1 This hardly seems strange, but there is reason why it should. The attempt to describe one language (in this case, music) in terms of the paraphernalia of another (speech) can lead to complications. The question needs to be asked whether speech is the only surrogate language available to the musicologist, or even whether it is the best one.
Charles Seeger first raised this question as early as 1939.2 It seems to have caused little stir at the time, and since no other modality had been put forward as a viable alternative—not even by Seeger, apparently—words remained central to the musicological function. By 1970 however it was becoming apparent to a few scholars that the verbal medium had begun to color the musicological act to an undesirable extent. Among the most evident proofs of this was musicology's inability to answer certain kinds of questions about music. While the musicologist might argue in many cases that it was never intended that his discipline be expected to do so, such evasive action hardly served to inspire confidence.
The kinds of questions still awaiting answers suggest the need for a different methodology, and it is unlikely that today's curriculum as presently constituted will ever come to grips with them. Among the issues begging resolution are these:
1) What, if any, are the real points of contact between Eastern and Western musical thought? (One would think the ethnomusicologists might be able to answer this one, but so far not one of them has been able to do so.)
2) Why does virtually all known music, ancient as well as recent, tend to be predicated upon the idea of the scale? (Since it is true that some "current" music, especially perhaps that kind involving the use of currents of an electrical sort, does not honor the scale principle but rather vitiates it, it might be wise to include a corollary to this question, as follows: what is the significance of attempts by some experimental composers today to break away from the time-honored scale idea?)
3) How does music relate, in the most ancient sense, to other aspects of man's cultural heritage such as government, religion, the calendar, medicine, and so on? (This is the sort of question most likely to be ignored by the ordinary classroom teacher, because it smacks of irrelevance. But who is to determine what is relevant to the study of music, and how is this to be determined?)
One of musicology's most pressing difficulties today is its inability to deal with earlier musical styles than those implied within the context of the Christian era. Although the study of ethnomusicology gives a broader perspective to the problem, it does not really solve it since the communicative methodology of the ethnomusicologist is, like that of the ordinary musicologist, primarily linguistic. Ethnomusicology adduces a sociological element not emphasized in the study of Christian art music; but while the connection between music and, say, political science may lie closer to the surface in some oriental cultures, the significance of such connections tends to be minimized as a rule.
The thought that words may not communicate as well as do numbersthe fundamental theoretic fabric of earlier musicis the basic tenet of a small group of musicians who are beginning to call themselves "mathematical musicologists." I fell into this discipline, quite unaware of its implications, as the result of a particular line of inquiry I was conducting into the history of the circle of fifths.3 At that time, any reference I was making to fundamental mathematic concepts was on so elementary a level (the idea of the circle, the principle of 12-base) that the revolutionary nature of the research simply was not apparent. However I was soon made aware of the work of a New York musician named Ernest McClain, and our communality of interests (as well, of course, as our separateness from what almost everyone else in the musical world seemed to be doing) engendered between us a lively correspondence.
McClain's specialty at the time of my first acquaintance with him centered upon the discovery of acoustic meanings in the dialogues of Plato. Much of this brilliant work was published during the early and middle 1970s.4 Gradually McClain branched out to include in his investigation a wide range of literary sources, from the Hindu  to the Bible.5 While the subject-matter of McClain's scrutiny has been early literature in almost every instance, the methodology is totally and uniquely mathematical. A McClain dissection normally convokes before the reader's eyes a bewildering maze of algebraic grids and diagrams. It is enough to intimidate the most stout-hearted. Nevertheless the accomplishments resulting from such a change in method bespeak the validity of mathematics as a proper language of musicological discourse. If McClain himself, most published to date of all mathematic musicologists on this side of the ocean, does not vouch for the unassailable integrity of all his diagrams, he has every reason to be regarded nonetheless as the leading figure in this emerging area. While space simply does not permit a lengthy consideration of McClain's work here, his statement with reference to the
to the Bible.5 While the subject-matter of McClain's scrutiny has been early literature in almost every instance, the methodology is totally and uniquely mathematical. A McClain dissection normally convokes before the reader's eyes a bewildering maze of algebraic grids and diagrams. It is enough to intimidate the most stout-hearted. Nevertheless the accomplishments resulting from such a change in method bespeak the validity of mathematics as a proper language of musicological discourse. If McClain himself, most published to date of all mathematic musicologists on this side of the ocean, does not vouch for the unassailable integrity of all his diagrams, he has every reason to be regarded nonetheless as the leading figure in this emerging area. While space simply does not permit a lengthy consideration of McClain's work here, his statement with reference to the  is characteristic: "What we are investigating . . . is . . . a realm of number theory in which music sets the problems, since musical patterns elevate certain numbers to a prominence pure number theory would not accord them."6
is characteristic: "What we are investigating . . . is . . . a realm of number theory in which music sets the problems, since musical patterns elevate certain numbers to a prominence pure number theory would not accord them."6
There is no better illustration of this position than some work recently done by Edith Borroff of SUNY at Binghamton. (See her article in this volume of Symposium.) Her computer project with respect to latent musical significance residing in the number 360 points to the propensity of certain numbers to survive culturally as "signs" or "artifacts," even if (in many cases) the original significance of the numbers has been lost. While words can change their meanings and pronunciations, there is something remarkably stable about a number. As an epistemological tool, such numbers as 360, the unlucky 13, even 2 when used as a number base (as suggested by Lévi-Straussian structural anthropology) cannot shed their fundamental arithmetical characters. A number always remains a number and never signifies any greater or lesser quantity. This essential integrity of number stands out as strongly to the archeoastronomer studying the significance of certain measurements at Stonehenge as to the musician seeking out possible acoustic meanings in favored Mesopotamian numbers like the sexagesimal number 360.
It is not difficult to see why man has set aside some numbers for special use. Man did not, presumably, create numbers, but he did in many cases create meanings for them. There is no evidence for believing that decimal metrication would be sweeping aside all other possible number-bases with such force and efficacy today if man had happened to have, for instance, eight instead of ten fingers on his hands.7 Similarly the importance of the number twelve over many thousands of years may be partly due to the fortuitous near-concurrence of the lunar and solar cycles. But twelve is also a very musical number. Evidence for the connection of music to astronomy in the primordially ancient mind has recently become especially strong. McClain writes,
As our study unfolds it will raise serious questions about the early development of mathematical thinking, about debts which the calendar and scale may owe to each other, and about the possible origins of both the mathematics of music and its related mythology. . . . Historians of science have barely begun to cope with certain kinds of material available to them, and we must await their judgment on many issues. A musical analysis of Rgvedic imagery will provide, we believe, a new tool for the study of the origins of science, of our calendar, of musical theory, and of the roots of our civilization.8
Obviously traditional musicological approaches will serve no purpose in exploring such connections as these. What then of mathematics—how does one employ it? It is apparent that scales in music, whatever their provenance and original significance, represent distances between tones measured proportionally. Mathematics can articulate the interlocking of these proportions and the nature of the intervals being compared. For example, in the musician's traditional "circle" of tones, normally generated by fourths and fifths, the distance characteristically marked off is that interval which can be loosely referred to as the half-step. In China this same unit is traditionally represented by the concept of the Lü's.9 The fact that the Chinese have recognized the circle of fifths since time immemorial ought to be a fundamental precept in the teaching of music history everywhere, because it is one of the true "universals" upon which the integratively minded scholar can draw. The fact that the Lü's are not so taught suggests the enormous chasm which still exists between practical ethnomusicology as taught in the United States and the reality it purports to represent.
If one is comparing fifths with octaves, a fundamental and disturbing mathematic truism comes to mind: powers of 2 (i.e., the octave) cannot ever coincide with powers of 3 (the fifth). This has been the stumbling-block upon which acoustically minded historians have failed in the past, but it need not concern the student who realizes that the ancients' awareness of such mathematical subtleties was far less acute than their sense of, or faith in, the essential unity of all observable phenomena.10 What a musician might call the comparative projection through musical "space" of octaves and fifths, a mathematician would label "2p3q," in which designation the letters "p" and "q" refer respectively to powers of 2 and 3. An interesting example of a 2p3q computer program, run in 1972 by the Sinomusicologist Fredric Lieberman, can be seen in Table 1.
TABLE I. THE LIEBERMAN PRINTOUT
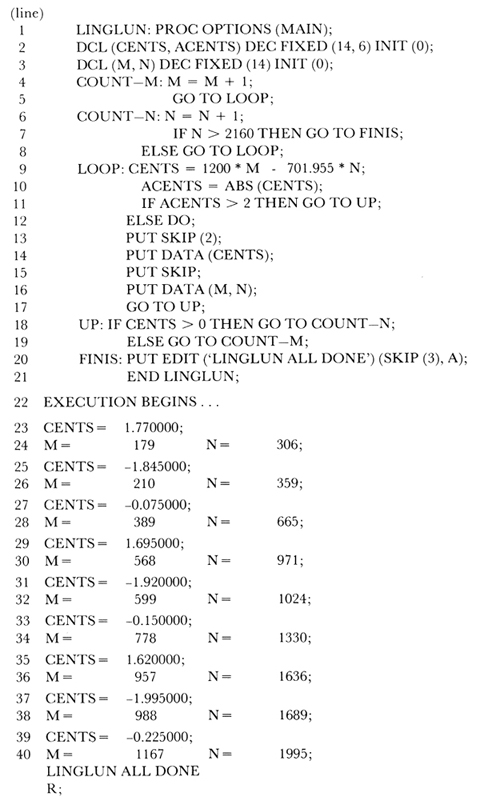
A useful aspect of this program is its inclusiveness. Lieberman had asked the computer (lines 7-13) to print any near-convergence between octaves and fifths to within a tolerance of two cents (in this table M refers to octaves, N to fifths).11 It is apparent (line 7) that Lieberman commanded the computer to stop at 2160 fifths. It is also apparent that no near-closures occurred between octaves and fifths before the already awe-inspiring span of 179 octaves/306 fifths. It is arresting to note in line 26 the near-coincidence of 210 octaves with 359 fifths. This is just one fifth short of the 360 with which Borroff became concerned four years later.
On the surface, the Lieberman printout would suggest that Borroff's hypothesis about 360 was little more than a gloriously apt near miss. The fact however was that Borroff hit the mark exactly and that the difference in readouts was due to a fundamental difference in the nature of the two programs. Since neither scholar was aware of the other's work, collusion is out of the question. The real difference is that Borroff's program was extremely specific: her only concern was to find out what a 2p3q projection might tell her about 360. The mathematic difference is shown in Table 2.
TABLE II.
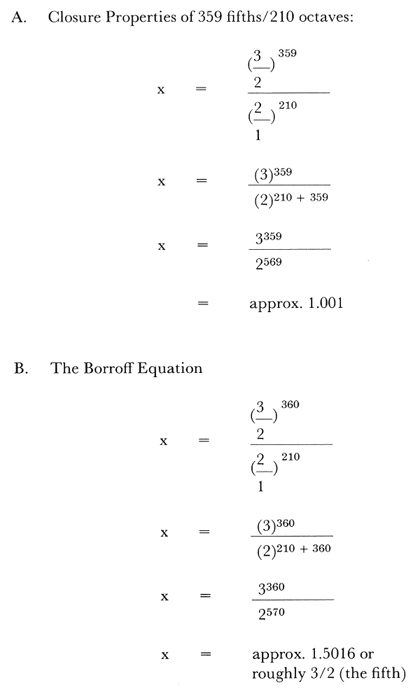
The Borroff program indicates that, while 30 circlings of a totally harmonic 12-note octave would result in 30 times 12 or 360 fifths, still harmonic at the octave, the fact is that because of the Pythagorean comma the point of near-concurrence is arrived at one fifth sooner. What one has at 360 is one additional fifth, nearly perfect in itself (just 16 thousandths too large) and made up of the accretion of 30 commas. The likely connection of this discovery with the motions of the heavenly bodies suggests why literally all high cultures since the Sumerians have venerated this "compromise" number, lying neatly at the intersection of 354-plus days (lunar year) and 365-plus days (solar year). It suggests one reason why the complicated sexagesimal numeration system with its all-time-high among number bases (60) played the enormous role it did in Babylonian science. Since 60 is the lowest common denominator between 10 and 12, the decimal and dozenal systems were able to survive side by side without serious conflict until comparatively recent time.12 Unfortunately, a need for homogenization within the mathematical and commercial worlds has now all but sounded a death-knell for "musicalized" dozenal methods.
The real problem with attempts to popularize mathematical musicology is its implicit return to the concerns, not of musica prattica vaunted among English-speaking peoples since Thomas Morley's Plaine and Easie Introduction of 1597, but of the old musica speculativa so carefully winnowed out of college music curricula by administrators over the past century. If the purpose for studying music is the training of able executants and nothing more, there is no place for mathematic techniques in music history. (A few scholars no doubt continue to hold to the position that knowledge ought to be worth pursuing for its own sake, however unpopular such an attitude seems to be these days.)
Word and Number conjure up two vastly different strains of human experience and intellection. To perceive verbally is not the principal motivation of modern science, but it is at the very core of primitive, mythic science and it continued to dominate the traditional university curriculum well into the twentieth century. To perceive mathematically is the province these days of the laboratory scientist, and the present-day truce between the humanist—the "man of letters"—and the scientist is an uneasy one at best.13 Since music normally identifies itself with the arts and humanities, reference to the use of number in musico-scientific discourse about music's history is destined to raise hackles. But in the categorization of academic disciplines by ancient and medieval scholars, music was placed not within the trivium of the word arts but rather in the quadrivium of disciplines essentially scientific by nature and ruled by number (fig. 1):
Fig. 1. THE SEVEN LIBERAL ARTS
| Number | Word |
| Arithmetic | Grammar |
| Geometry | Rhetoric |
| Astronomy | Logic |
| Music |
While it can be argued that this reflected merely the speculativist theoretical view and not that of the pragmatist, it can also be construed to show that, the farther one ventures backward in time, the more words prove an inadequate measure of the musical impulse and numbers come to the fore. The instrumental musician still measures his art numerically today; the singer, proverbially (whether accurately or not) insouciant about counting and measuring, hews to a different line: the text. Musical scales come readymade on many if not most musical instruments; they never come naturally to singers. It is not too surprising that, among the ranks of scholars most interested in mathematical musicology today, it is easy to find horn players, clarinetists, keyboardists, and other instrumentalists. It is much more difficult to find singers.
It is true that music was not always welcomed among the Number sciences, even by early thinkers. To the Greeks máthema had very specifically didactic connotations, meaning "that which is learned," or a "lesson."14 The number of disciplines included varied from three (arithmetic, geometry, and astronomy) to four (those listed plus music, or harmonics). This somewhat uneasy relationship of music to the mathematic sciences apparent even in classic times continued until it was dissolved completely during the late Renaissance and early Baroque. Christian clerics noted what was to them a principal difference between music and the other sciences: it only could convey moral values. Today the acoustician and writer Siegmund Levarie finds musicologists by and large to be "instinctively afraid of numbers."15
If there is truth in what Ernest McClain has to say about acoustic implications in the ancient  , or Edith Borroff about the extraordinary ramifications of the number 360, traditional music historiography is certainly due for a much-needed reappraisal. But mathematical musicologists at the same time need to come out of the woodwork and be counted. To judge from typical college catalogs (and this includes the largest music departments just as surely as the smallest), there is no such thing as mathematical musicology. A second problem has to do with communication, since most mathematical musicologists are forced to work in isolation and may even be duplicating one another's research (as happened to some extent with Borroff and Lieberman).
, or Edith Borroff about the extraordinary ramifications of the number 360, traditional music historiography is certainly due for a much-needed reappraisal. But mathematical musicologists at the same time need to come out of the woodwork and be counted. To judge from typical college catalogs (and this includes the largest music departments just as surely as the smallest), there is no such thing as mathematical musicology. A second problem has to do with communication, since most mathematical musicologists are forced to work in isolation and may even be duplicating one another's research (as happened to some extent with Borroff and Lieberman).
If traditional musicologists feel intimidated by references to musical number, they will be reluctant to admit newer research and teaching techniques on parity with their own. University administrators able to justify performance and performance-related subjects in music will tend to be coolly suspicious of any return to older, speculativa attitudes smacking on the surface of numerology and number mysticism. This is little short of ironic, since it was once these same Pythagorean attitudes, relating music to number, which found a sympathetic haven in the academic world.
Modern-day Pythagoreans are not numerologists nor mystics. They are practical, hard-working historians, theorists, and estheticians, who have seen once again a place for number in the world of music. This may alarm colleagues who view their art in quite a different way. But a university's first obligation is to its students, who presumably deserve truth above all else. Man's understanding of the truth changes from one century to another and even from one decade to the next. Can this nation's colleges and universities manage to make the change?
1Charles Seeger, "Systematic and Historical Orientations in Musicology," Acta Musicologica XI (1939), p. 126. In this passage, Seeger calls musicology a "linguistic discipline."
2See ref. 1 above. Also Charles Seeger, Studies in Musicology, 1935-1975 (University of California Press, 1977), p. 7.
3Fred Fisher, "The Yellow Bell of China and the Endless Search," Music Educators Journal LIX, 8 (April 1973), pp. 30-33, 95-96, 98. North Texas State University research grant (1971-72): "The Musical-Circle Concept in Ancient Thought."
4Principal among McClain's articles from 1973 to 1976:
"Plato's Musical Cosmology," Main Currents in Modern Thought XXX, 1 (September-October 1973), pp. 34-42.
"Musical 'Marriages' in Plato's Republic," Journal of Music Theory XVIII, 2 (Fall 1974), pp. 242-268.
"A New Look at Plato's Timaeus," Music and Man I, 4 (1975), pp. 341-360.
"The Tyrant's Allegory," Interdisciplina I, 3 (Spring 1976), pp. 23-37.
5See especially Ernest McClain, The Myth of Invariance (N.Y.: Nicolas Hays Ltd., 1976).
6The Myth of Invariance, p. 4.
7David E. Smith, History of Mathematics I (N.Y.: Dover, 1958 reprint of the Ginn and Company edition), p. 10.
8The Myth of Invariance, pp. 3-4. McClain's principal interest tends to focus upon the rise of principles of Just intonation in human thought. Mathematicians would describe this tuning as 2p3q5r. Nevertheless McClain's catholic concern with any tuning system discernible through philological methods is also frequently expressed, as in this remarkable sentence in the Main Currents article: "Thus the Republic may be considered our oldest extant treatise on equal temperament" (p. 38).
9See for instance the writings of Fritz Kuttner in Ethnomusicology from volume 8 onward, and my article "The Yellow Bell of China," ref. 3 above.
10This point was discussed in the first few issues of my research newsletter Circle Comment (1971-72). While issues 1-20 are now regrettably out of print, a few indexes and copies of later issues remain available.
11Cent: a logarithmic unit devised by Alexander Ellis, 1200 to the octave, 100 to a single equal-tempered halfstep.
12The Chinese were very ambivalent about this, merging 10- and 12-base systems in their calendar. See Dirk Struik, A Concise History of Mathematics, 3rd edition (N.Y.: Dover, 1967), p. 32; Joseph Needham, Science and Civilisation in China III (Cambridge University Press, 1959), p. 396.
13This dichotomy was described by C.P. Snow in 1959 as the "Two Cultures" syndrome. See C.P. Snow, The Two Cultures and a Second Look (Cambridge University Press, 1969).
14Liddell-Scott-Jones, Greek-English Lexicon (Oxford: Clarendon Press, 1968).
15The Myth of Invariance, preface by Siegmund Levarie, p. xi.


