Reducing a folk melody to its pitch spectrum does not fully elucidate its development, and few participants in an oral tradition consciously follow scalar dicta. Nonetheless, an awareness of the tonal material of a folksong is essential to the broader understanding of the idiom that that song represents. Much confusion has arisen from the varied ideas concerning the anhemitonic1-pentatonic basis of most British-American2 folksongs. What now is needed is an approach that is basically simple yet capable of accounting for the often complex tonal character of the tunes. The offering of such a solution here is preceded by a short summary of select earlier views.
Annie Gilchrist conducted one of the first significant investigations of pitch in British folksong. Acknowledging earlier observations by Parry, Culwick, and Helmholtz, Gilchrist concluded that many highland Scottish melodies are rooted in the five scales given in Figure 1.3
Figure 1. The five pentatonic scales of Scottish folksong, according to Gilchrist. The regular members of the scales are shown as white notes, while auxiliaries are shown in black.

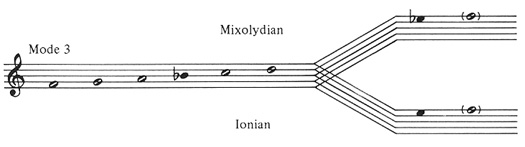
Gilchrist also noted that several heptatonic modes could be formed by the insertion of auxiliary tones into the minor thirds of the pentatonic scales (Figure 1, above). The tone that Gilchrist designated b-flat was fixed, while e was variable.4 In her Mode 3, the downward inflection of e would throw an Ionian melody into the Mixolydian mode. Conversely, a Mixolydian tune could contain one or more Ionian leading tones (Figures 2 and 3).
Figure 2. Table of pentatonic and heptatonic modal equivalencies, after Gilchrist.

Figure 3. Formation of either the Mixolydian mode or the Ionian mode through inflection or non-inflection of the auxiliary e in Mode 3.
In his study of southern Appalachian folksong, Cecil Sharp concurred with Gilchrist's pitch designations, her ordering of the pentatonic scales, and her idea of a kinship between the pentatonic and heptatonic series. However, he found that the auxiliary e was fixed, while the b was variable—the opposite of what Gilchrist had discovered in the Scottish tunes (Figure 4).5
Figure 4. The pentatonic series of Sharp and Gilchrist, with auxiliary notes.

Acting upon his observations of pentatonic and heptatonic modal similarities, Sharp also propounded a hypothesis of scalar evolution from primitive, mostly monotonal, chant through tetra-, penta-, and hexatonic melodies, and thence to tunes using heptatonic modes and ultimately the modern scales.6 This idea soon became popular, and many similar concepts emerged until around mid-century.7
Investigators after Sharp discovered that British and British-American folksong scales were more numerous and diverse than Gilchrist and Sharp had suspected. By ca. 1960 approximately fifty different scales had been found, and much effort was being made toward classifying them. One such procedure, developed by George Foss,8 begins with Bartók's practice of associating all known folksong scales with a common gamut.9 In Foss's system, each note of the gamut is assigned a number (Figure 5),10 and alterations of pitch within a melody are indicated by the appropriate chromatic signs (Figure 6).11
Figure 5. The gamut used by Foss, with its tones numbered after Bartók.

Figure 6. Foss's encoding of a folksong scale with chromatic alterations.

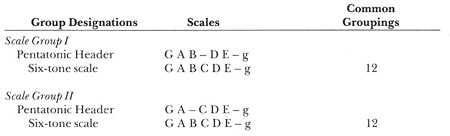
An encoded scale is assigned by its pitch letters to one of five "scale groups." Each group is headed by a pentatonic series12 and consists of all known six-and seven-tone scales that can be formed from it. The commonality of a scale to two or more groups is indicated by an additional code. For example, a member of both Group I and Group II is earmarked 12 (Figure 7).13
Figure 7. A scale common to two groups, adapted from Foss's scalar table.
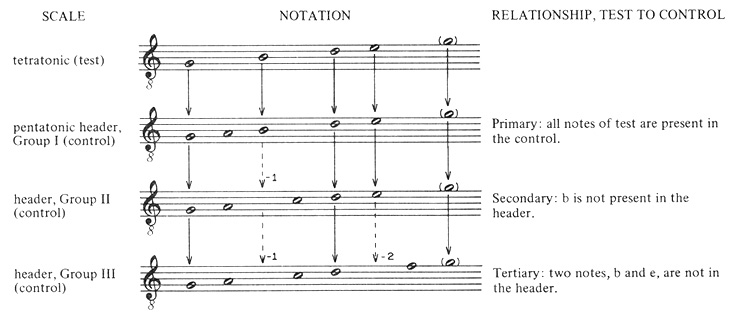
Finally, Foss observes some less common tetra- and pentatonic aggregates that are related to two or more of the standard pentatonic scales. These kinships are described as primary, secondary, or tertiary, according to how many pitches of the irregular scale also occur in the header (Figure 8).14
Figure 8. Foss's classification of the tetratonic scale g-b-d-e-g' in terms of its relationships to three pentatonic headers.
Bronson's mid-century approach reflects Sharp's earlier views by its focus on the relationships between the pentatonic series and the hexatonic and heptatonic scales and modes. Bronson recognizes many other scales, but he attributes them to ad libitum inflections of regular scalar degrees. The core of his scalar theory is illustrated by the quaintly charming "Chart of Modal Correspondences," or mode-star (Figure 9).15
Figure 9. The Bronson mode-star.

The heptatonic modes are shown at points around the perimeter of the star. Hexatonic scales, shown at the points where the rays converge, are said to be modal hybrids, each with one missing heptatonic degree.16 Pentatonic scales, indicated by π, are situated within the star.17
The second half of this century has been marked by a general drift away from evolutionary concepts and postulations of large, complex scalar families. As an alternative to such ideas, Cazden sets forth a broad method of classification based upon the prevailing tonality of a melody.18 He also maintains that such factors as the raised (Ionian) and lowered (Mixolydian) seventh, the raised (Dorian) sixth, and the lowered (Phrygian) second should be treated as "chance variants" of major and minor scales.19 Cazden further contends that a tonally ambiguous tune represents a confluence of two broad tonalities. To cite an example, many Aeolian, or minor, folktunes contain transient modulations that involve the downward shifting of the key center into the Mixolydian mode, which Cazden describes as simply major. He calls this phenomenon the "subtonic juncture" (Figure 10).20
Figure 10. Application of Cazden's theory of the subtonic juncture.

The present theory of southern uplands scales draws upon the views summarized above, but much older ideas are taken into account as well. Despite archaic regional terminology, the information in surviving documents points toward the existence of a sophisticated, codified, and probably pentatonic scalar system in Britain by the close of the Middle Ages. Part of this system continued to govern indigenous practices until modern times. From these practices and the written material we can reconstruct what once may have existed.
Particularly important is a gloss by Lewis Morris (1700-1765), in British Library Manuscript Additional 14905.21 Morris copied part of this gloss from an older document of unspecified date. Translated from the Welsh, this portion reads:
There are five established and warranted keys; and out of those may be formed other keys at pleasure.
One finger of a Crythor [crwth-, or lyre-, player] . . . keeps three keys; namely the lower key, the sharp key and the flat key; and this is the long finger. The fore finger keeps the intermediate key and the mixt key; these are the five principal keys. . . .
These are the . . . canons of instrumental music, all regulated by principle, as they were composed in a congress, . . . in the time of Grufydd [sic] ab [sic] Cynan [ca. 1055-1137].22
Flat key (lledyf gywair) reappears, in a hand other than Morris's, on manuscript page 108, as a label for a harp scale. Like the others on the page, this scale is written in letter notation and is paralleled by a companion scale that now is thought to be a scordatura. Figure 1123 shows the flat key (white notes) and its pentatonic equivalent (black notes).
Figure 11. Lledyf gywair and its scordatura.

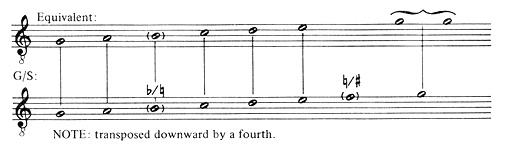
The scordatura of lledyf gywair corresponds intervallically to Gilchrist and Sharp's Mode 1, except for the auxiliary seventh above the keynote (Figure 12).
Figure 12. The pentatonic equivalent of lledyf gywair, with Gilchrist and Sharp's Mode 1.
Thus a connection is established between a modern folksong scale and a seventeenth-century one, and the latter was nominally associated with a scale that may have been in use by ca. 1400. Gilchrist and Sharp's observation of five pentatonic scales lends a measure of strength to this somewhat tenuous link between the most recent and earliest-known Celtic scales (the "five established and warranted keys").
In light of what is found in MS Robert ap Huw, it might be wise to think of British-American folksong scales in pentatonic rather than heptatonic terms. This dispenses with the idea of missing heptatonic degrees in favor of one that views these notes as additions to the pentatonic series. Figure 13 shows the modes of Gilchrist and Sharp, with Mode 1 beginning on g, in conformity to the practice established by Bartók.
Figure 13. Transposition of the complete series of Gilchrist and Sharp, with Mode 1 beginning on g.

The scales are offset to show their interrelationships through successive inversions. Figure 14 is a condensation of the pentatonic series into a gamut comprising five overlapping, interlocking segments.
Figure 14. Figure 13 condensed into a gamut.
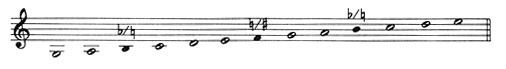
Figure 1524 shows the gamut extended to encompass the maximum range of all melodies combined.
Figure 15. The pentatonic series with its variable auxiliaries (black notes) and numerical pitch codes.

The present system, like those of Bartók and Foss, uses numerical designations for the pitches, as shown in Figure 15, but the numbering here differs considerably from that used in the earlier procedures. The new nomenclature functions as follows:
1) The regular members of the gamut are numbered 0 through 4, for g, a, c, d, and e, respectively.
2) The auxiliaries, b and f, are numbered 5 (b) and 6 (f).25
3) These pitch-class codes occupy the units' place in the complete three-digit label.
4) An unaltered tone is indicated by a zero in the tens' place of the label. A 1, in the tens' place indicates a sharp, while a 2 denotes a flat.
5) Pitches in the central octave of the gamut (g through
') are indicated by a zero in the hundreds' column. Higher notes are shown by a 1 in that column, and lower notes are numbered in the 200s. For instance, the complete code of g is 000, that of g' is 100, and that of G is 200.
This coding was developed to expedite both the manual and the mechanical processing of data in tune-analysis and -comparison. Therefore pitch codes are entered into textfiles, on floppy disks, in their shortest forms. For example, c' is filed as 2, not 002, and 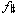 ' is filed as 16, not 016. In each of these cases, the complete label would occupy more than the necessary amount of filing space, since the computer ignores prefatory zeroes when it converts a decimal value to a binary one. The complete code was found to be beneficial in certain manual operations, and for this reason it does appear on written data sheets.
' is filed as 16, not 016. In each of these cases, the complete label would occupy more than the necessary amount of filing space, since the computer ignores prefatory zeroes when it converts a decimal value to a binary one. The complete code was found to be beneficial in certain manual operations, and for this reason it does appear on written data sheets.
For filing, analysis, and comparison to other melodies, a pentatonic tune is transposed to its proper position in the common gamut according to the following criteria:26
1) the levels of minor thirds that occur within the tune and, in transposition, correspond to the minor thirds that are found within the common gamut whenever its auxiliaries are omitted;
2) the predominant tonality of the tune—a criterion which normally is met if the first one is correctly applied; and
3) the locations of the auxiliary tones within the transposed tune. The melody is best transposed so that most (or, ideally, all) auxiliaries occur either on weak beats or on afterbeats—and are not essential to the definition of the tune's tonality.
Pentatonic melodies with major tonality are aligned with the c-segment of the gamut, and c usually functions as the keynote (Figure 16).27 The g-segment is not used for Mixolydian tunes because it lacks the distinguishing major third above g unless the auxiliary b is used.
Figure 16. Transposition of a "Barbara Allen" tune to its proper level in the common pentatonic gamut. Asterisks denote minor thirds that determine the alignment.

Minor-pentatonic melodies are aligned with the a-segment, and a is the usual keynote. The d- and e-segments are not used for Dorian and Phrygian melodies. Without the auxiliary f, the d-segment lacks the characteristic minor third above the normal tonic. If the auxiliary b is not used, the e-segment lacks the fifth above the key-note, and this degree is rarely absent from folk melodies.
Tunes with no clearly pentatonic properties (i.e., no minor-third determinants) are treated as either major- or minor-pentatonic melodies with auxiliaries. Their keynotes usually are either c or a, according to the principle of prevailing tonality, but two additional conditions must be met whenever pentatonicism is not patent. They are as follows:
1) Auxiliary notes should not be used as major or minor thirds above key-notes, and they should not sound on strong beats.
2) Notes that transpose as sharped and flatted notes should occur, whenever possible, as chromatically altered auxiliaries (first choice) or commonly inflected degrees (second choice). The latter are thirds and sevenths and, to a lesser extent, seconds and sixths above the keynote.28
The transposition of the familiar melody of "Old Joe Clark" (Figure 17) illustrates the relocation of a non-pentatonic, tonally major melody.
Figure 17. "Old Joe Clark" (middle staves), transposed incorrectly (upper staves) and correctly (lower staves). The auxiliary notes are circled.

Both the range of the tune and the Mixolydian seventh might first suggest g as the keynote, but this would involve the occurrence of the auxiliary b on the strong initial beats of measures in two instances (measures 4 and 8, upper staves). Also, the use of b as the major third above g is improper. Beginning and ending the melody on c establishes a regular gamut member (e) as the major third above the keynote. The use of b-flat as the Mixolydian seventh is permitted under Condition 2. Finally, all auxiliaries meet Condition 1 if the tune is aligned with the c-segment.
Occasionally both the original keynote (and hence the transposed one) and the tonality are unclear. In Figure 18,29 for example, the original keynote could be either f (= c in the common gamut) or d (= a); and the tonality is frequently ambiguous, most notably at the final cadence.
Figure 18. Hymn-tune, "The Hebrew Children."

One could even make a case for a (= common-gamut e) as the keynote, inasmuch as it opens, closes, and occurs most frequently within the tune. In cases such as this, the level of pentatonic minor thirds becomes the sole criterion for determining the relationship between the tune and the gamut (Figure 19).30
Figure 19. Transposition of "The Hebrew Children" to the common gamut on the basis of minor-third location.

It should be stressed that the present theory does not discount the normal prominence of one tone over the others in a tune. Keynote ambiguity is the exception rather than the rule, yet it happens often enough that keynote-assignment in this procedure is not a strict declaration of tonic or scalar alliance. The proper alignment of the intervals (especially the minor thirds) of the tune with those of the entire gamut is more important than determining the keynote.
One other trait of pentatonic folktune behavior needs to be pointed out. This is the gamut-shift, or the relocation of the gamut at a different tonal level, usually around a short series of adjacent notes that are common to both levels. "Black is the Color of My True Love's Hair" (Figure 20) immediately reveals its ties to the second segment of the common gamut, yet the prominence of the auxiliary b throughout the tune (bracketed and circled notes) is difficult to explain according to new theory presented thus far.
Figure 20. "Black is the Color of My True Love's Hair," with brackets under the motifs using the auxiliary b (circled).

What seems to be taking place is the intermittent crystallization of the gamut around the series g-a-b, which can be extended to form the scale g-a-b-d-e. This equivalent of the third common-gamut segment, c-d-e-g-a, is the basis of the relocated gamut, of which b is a regular member instead of an auxiliary (Figure 21).
Figure 21. Shifting of the common gamut (C) to another level (R: relocated).

In the reversal of this process, b loses its status as a regular gamut member and becomes an auxiliary again as the gamut recrystallizes around g-a-c. Figure 22 illustrates the reestablishment of the common gamut,
Figure 22. The return of the gamut to its original level (C).

and Figure 2331 shows the gamut-shifts in the tune in question.
Figure 23. Figure 20, with all gamut-shifts shown.

This theory of gamut-shifting is similar to Cazden's theory of the subtonic juncture. It differs from the latter in that it explains melodic events in wholly melodic terms, while Cazden's theory entails the presence of harmonic implications in the tune. Such implications are easily detected by the musician who has been trained, either formally or informally, in harmonic practices, but their perception by all folksingers of previous generations is questionable.32 In contrast, it seems that these earlier practitioners were keenly aware of the pentatonic gamut, even though this awareness was not a fully conscious one in most cases. Therefore what Cazden explains as a movement of the implied harmonic framework might be better explained as a movement of the expressed melodic framework.33
In conclusion, it seems that melodic pentatonicism reached a high level of development in Celtic Britain prior to its supplantation by heptatonic modality. Many folktune traits based upon this pentatonicism have survived until modern times in remote areas. Early outside observers noted the principal traits as they were revealed in songs. Subsequent attempts to account for the many complexities of the system have frequently obscured its fundamentally simple foundation. That foundation is a series of five overlapping octave divisions that form a gamut. Melodies move within the gamut, often but not always with single tones as their centers of melodic gravity. Unlike the heptatonic tonal or modal melody, whose tonic- and final-identities are of great importance, the pentatonic tune is best explained and understood in terms of how its intervals relate to those of the entire gamut. Many folktunes utilize optional, or auxiliary, gamut tones and thus are not wholly pentatonic. However, most of them reflect the influence of this scalar system whose flexibility is as impressive as the tenacity of its main characteristics.
1Anhemitonic: characterized by the presence of no interval smaller than a whole step when only the regular scalar tones are used. Half steps appear in some melodies that contain additional non-standard, or auxiliary, tones. The temperament of any interval depends upon performer, locale, and other factors.
2In this study, "British" refers to the British Isles in general. Neither "Anglo-American" nor "Celtic-American" is a consistently correct label for southern uplands folksong. Therefore "British-American" will be used whenever a reference to Insular origin is apropos.
3Annie G. Gilchrist, "Notes on the Modal System of Gaelic Tunes," English Folk Dance and Song Society Journal 4 (December, 1911):150-53.
4Ibid., 151-53.
5Olive Campbell and Cecil J. Sharp, English Folk Songs from the Southern Appalachians (London: Putnam, 1917), xv-xviii; Gilchrist, "Gaelic Tunes," 151-53.
6Campbell and Sharp, English Folk Songs, xv-xvi.
7See, for instance, Joseph Yasser, A Theory of Evolving Tonality (New York: American Library of Musicology, 1932), and John Vincent, The Diatonic Modes in Modem Music (Berkeley: University of California Press, 1951).
8Roger Abrahams and George Foss, Anglo-American Folksong Style (Englewood Cliffs: Prentice-Hall, 1968).
9Bela Bartók, Hungarian Folk Music, trans. M.D. Calvacoressi (London: Faber & Faber, 1932). Much of Bartók's methodology was first proposed by Ilmari Krohn, in "Welche ist die beste Methode, um Volks- und volksmässige Lieder nach ihrer melodischen (nicht textlichen) Beschaffenheit lexikalisch zu ordnen?" Sammelbände der Internationalen Musikgesellschaft 4 (1903):640-60.
10Abrahams and Foss, Anglo-American Folksong Style, 211.
11Ibid., 214.
12The pentatonic headers correspond intervallically to Gilchrist and Sharp's modes, but Foss begins each of them on g and then arranges them in alphabetical order.
13Abrahams and Foss, Anglo-American Folksong Style, 211.
14Ibid., 217.
15Bertrand Harris Bronson, The Ballad as Song (Berkeley: University of California Press, 1969). This volume is a collection of select Bronson studies on the Child Ballads. The mode-star appears on p. 85; used by permission.
16For example, the scale c-d-e-f-g-a-(c) is, according to Bronson, a confluence of the Ionian and Mixolydian modes, with the seventh degree (either b or b-flat) missing. This is encoded in Bronson's procedure.
17For example, the first pentatonic scale, π1, is c-d-e-g-a-(c), and it is formed by dropping the fourth and seventh degrees from the Ionian mode. It is encoded 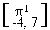 . This scale is placed first in Bronson's pentatonic series because of its similarity to the Ionian mode, which Bronson reports to be the most common heptatonic folktune scale.
. This scale is placed first in Bronson's pentatonic series because of its similarity to the Ionian mode, which Bronson reports to be the most common heptatonic folktune scale.
18I.e., whether the third above the keynote is major or minor. See Norman Cazden, "A Simplified Mode Classification for Traditional Anglo-American Song Tunes," Yearbook of the International Folk Music Council (1971):44-77.
19Ibid., 68.
20Ibid., 65-66.
21Also known as MS Robert ap Huw, after its principal owner, this codex contains harp etudes and various annotations dating from the early seventeenth century. It also includes material copied from a sixteenth-century book that had belonged to William Pennlyn, a harpist in the Tudor court. Morris's most significant additions are found on MS pages 3-8.
22"Of the Welsh Music," Cambrian Register (1795), 387-89. This translation was checked, with the assistance of Nansi Richards and Alun Davies (1972), using a printed transcription in Owen Jones, ed., Myvyrian Archaiology [sic] of Wales, 3 vols. (London: Rousseau, 1807), 3:438. There is no support for the claim concerning the origin of the information in the time of Gruffydd ap Cynan. The continuous cultural contact between Ireland and Wales from ca. 1400 suggests this as a more likely date.
23After Owen Jones, Myvyrian Archaiology of Wales, 3:446-47. See also Osian Ellis, "ap Huw, Robert," The New Grove Dictionary of Music and Musicians, 20 volumes, ed. Stanley Sadie (Washington, D.C.: Grove's Dictionaries of Music, 1980), 1:501-2. Ellis kindly communicated his conclusion to me in 1972 while the article on Robert ap Huw was in preparation.
24J. Marshall Bevil, "Centonization and Concordance in the American Southern Uplands Folksong Melody: A Study of the Musical Generative and Transmittive Processes of an Oral Tradition" (Ph.D. diss., North Texas State University, 1984), 82.
25The occurrence of these auxiliary tones provides part of the argument in defense of the evolutionary theories of Sharp and others, and the gloss in MS Robert ap Huw briefly mentions "recess notes" (MS, p. 4). However, if these were the auxiliary gamut members, then all that can be safely concluded is that Insular pentatonicism was being influenced by heptatonic modality at the close of the Middle Ages. There is no evidence to support assumption of either a perceived inferiority of pentatonicism or a process of species betterment through the exclusion of pentatonic tunes.
26In the current procedure, a common gamut is used for all melodies because tune-comparisons are done by computer on a note-by-note basis, and points of intervallic concordance will not be correctly detected unless they share the same pitches. Further, such transposition greatly facilitates the manual aspects of analysis.
27C. Sharp, English Folk Songs from the Southern Appalachians, 2d ed., ed. Maud Karpeles, 2 vols. (London: Oxford University Press, 1932), 1:195; Bevil, "American Southern Uplands Folksong Melody," 83.
28B. Bronson, "Are the Modes Outmoded?" Yearbook of the International Folk Music Council (1972):24-25. This essay was written in reply to Cazden's criticism of modal terminology and analytical procedures, in "A Simplified Mode Classification."
29The Sacred Harp, 3rd ed., ed. Benjamin Franklin White and E.J. King (Philadelphia: Collins, 1860), 61.
30Bevil, "American Southern Uplands Folksong Melody," 88.
31Ibid., 74.
32Early twentieth-century reports of unaccompanied solo singing are numerous. The occasional use of instruments was governed by the tradition of the immediate area. Sharp (English Folk Songs, 2d ed., xxvii) informs us:
I came across but one singer [out of 281 informants] who sang to an instrumental accompaniment, the guitar. . . . Mrs. [Olive] Campbell . . . tells me that . . . singers occasionally play . . . the dulcimer.
Concerning the dulcimer, Karpeles comments (Sharp, English Folk Songs, xviii):
The dulcimer . . . we saw and heard only in some of the Kentucky mountain schools and never in the homes of the people, where it is evidently but rarely to be found.
33An earlier concept of melodic modulation is Kurt Reinhard's theory of the third-second nucleus. Such a pattern may be either a melodic third and a melodic second overlapped or a second and a third in conjunct juxtaposition. According to the theory, a folk melody is constructed around a succession of these nuclei, and modulation is accomplished when two overlapping series are adjacent to each other, as shown:
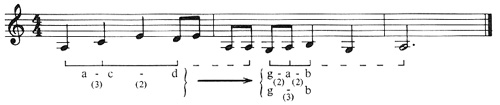
See K. Reinhard, "On the Problem of Pre-Pentatonic Scales, Particularly the Third-Second Nucleus," Journal of the International Folk Music Council 10 (1958):15-17.


